Classroom Observations
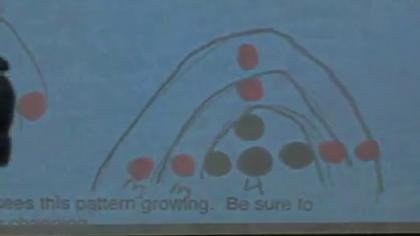
Teachers who are developing students’ capacity to "attend to precision" focus on clarity and accuracy of process and outcome in problem solving. A middle childhood teacher might engage his students in a "number talk" in which students use an in/out table and a plotted graph to "guess [the teacher’s] number." An early adolescence teacher might distribute cards with different symbol strings to his students, asking them to mingle to group and categorize their symbol strings, explaining and defending their groupings. A teacher of adolescents and young adults might continually probe her students to defend whether their requirements for a particular quadrilateral will always be the case, or whether there are some flaws in their group’s thinking that they need to refine and correct. Visit the video excerpts below to view multiple examples of teachers engaging students in attending to precision.
The Standard
Mathematically proficient students try to communicate precisely to others. They try to use clear definitions in discussion with others and in their own reasoning. They state the meaning of the symbols they choose, including using the equal sign consistently and appropriately. They are careful about specifying units of measure, and labeling axes to clarify the correspondence with quantities in a problem. They calculate accurately and efficiently, express numerical answers with a degree of precision appropriate for the problem context. In the elementary grades, students give carefully formulated explanations to each other. By the time they reach high school they have learned to examine claims and make explicit use of definitions.
show more